Neutrino production in proton-proton collisions supports the rotating lepton model

Direct observation of neutrino production in proton-proton (pp) collisions at CERN LHC lends strong support to the Rotating Lepton Model, Constantinos G. Vayenas¹, Dionysios Tsousis^1,2 and Eftyhia Martino¹ (1University of Patras, ²Stanford University) tell us

Understanding the internal structure of hadronic composite particles, such as protons and neutrons and computing their masses from their constituent elementary particles, such as neutrinos and other leptons, has been a long-sought goal in Particle Physics, a goal of central importance for enhancing our understanding of our Universe.

The two groundbreaking CERN experiments and the RLM

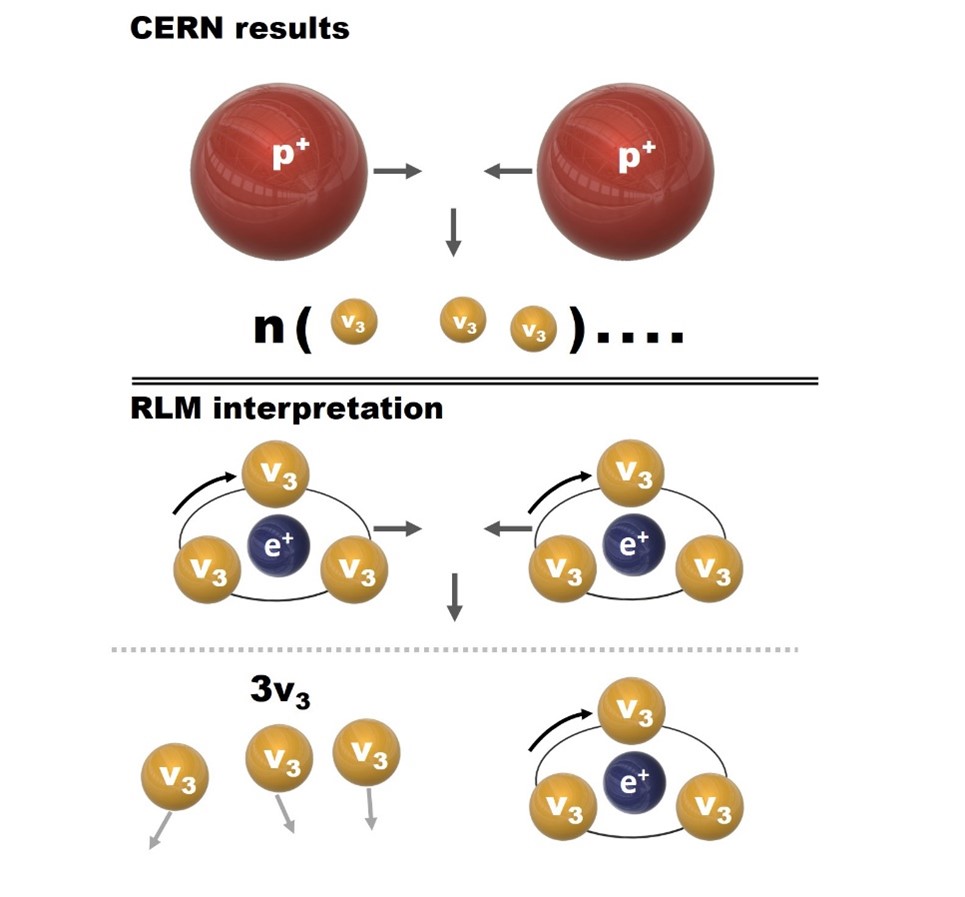
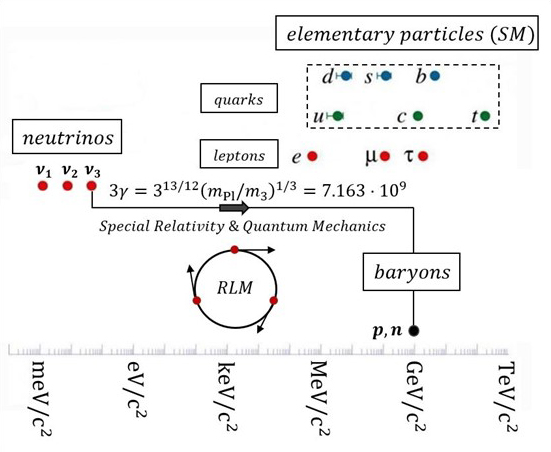
The recent pioneering findings at CERN LHC (Fig. 1) ^(1,2) have shown that proton-proton collisions lead to the production of neutrinos, which are the lightest of all particles with masses a hundred billion [1011] times smaller than the proton mass. ⁽³⁾

This can be understood directly ^(4-6) via the Rotating Lepton Model (RLM) of composite particles ^(4-6), which describes protons as triangular gravitating neutrino structures which rotate around a central positron and describes neutrons as the same structure after losing its central positron (Fig. 2).

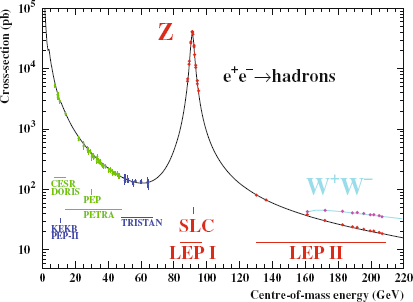
A second equally groundbreaking but until recently not fully understood experimental observation, also originating from CERN ⁽⁹⁾, are the results of the pioneering positron-electron annihilation (PEA) experiments ⁽⁸⁾ (Fig. 3), which produce huge amounts of the Z boson (Fig. 3). These fascinating results can also be immediately understood via the RLM ⁽¹⁰⁾ which has shown that the Z bosons are rotating positron–electron- neutrino triads. ⁽¹⁰⁾ The lifetime of these triads is very short, i.e., of the order of one revolution. ^(3,4)

Therefore, it is now becoming obvious that the Z bosons act as catapults for generating ultrarelativistic neutrinos. This constitutes the first and crucial step for baryogenesis. ^(10,11)

The mechanism of hadronization (or baryogenesis)

Careful examination of the decay products of several hundreds of composite particles, such as of hadrons (including baryons and bosons) ^(9,12), has shown that the ultimate decay products of all composite particles are only the following five leptons: The electron [e^–], the positron [e⁺] and the three neutrinos [ν₁, ν₂ and ν₃], the masses of which were first measured by Kajita ⁽³⁾ and McDonald ⁽¹³⁾ in their pioneering work. These masses are of the order of 3 to 50 meV/c², i.e., 12 orders of magnitude (a trillion times) smaller than the masses of electrons [10⁶ eV/c²] and protons/neutrons [109 eV/c²].

A first question arising from these extremely demanding to measure and dramatically different mass values of neutrinos vs those of the basic constituents of atoms (i.e., protons, neutrons and electrons), is how the tiny masses of neutrinos are related to the huge (by neutrino standards) masses of our familiar electrons and protons which are typically 11 orders of magnitude bigger than those of neutrinos.

The answer to this question is obtained by the ingenious 1905 masterpiece of Einstein, i.e., by Special Relativity, which, in simple words, dictates that what is really important in this world is not what we measure about ourselves but instead what others observe about us.

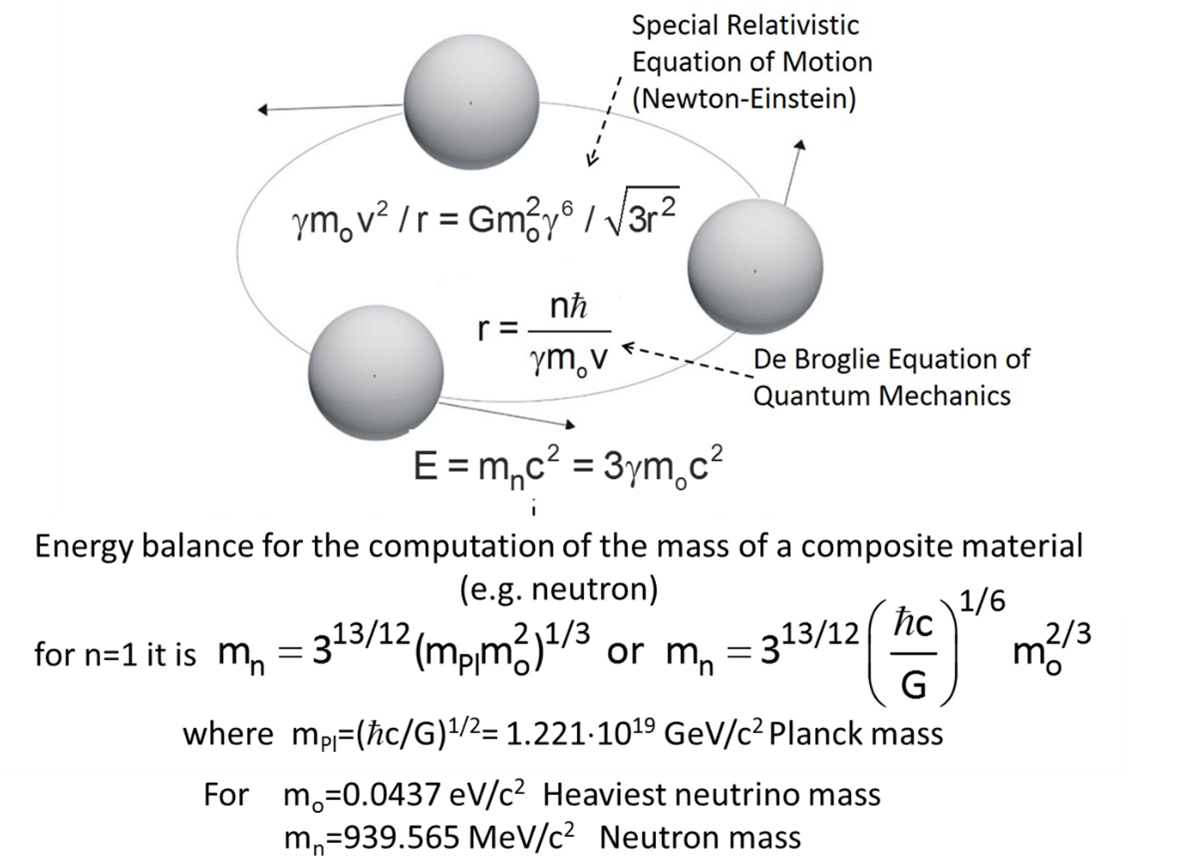
Thus, the measured relativistic mass, mo, of a particle, e.g., a neutrino, of rest mass mo , travelling with a Lawrence factor of 1010 is of the order of 10⁹ eV/c², i.e., 1 GeV/c², i.e., of the order of proton and neutrino masses. ⁽¹²⁾ [1]. At the same time, its gravitational mass, 3mo ^(8,9) reaches the Planck mass. ^(7,12). This provides the key idea of the RLM of composite particles, a simple Bohr-type model of three gravitating rotating neutrinos moving with speeds close to the speed of light ^(4-6). This appears to be how the matter of our Universe has been made ^(5,7) (or is being made even today according to the pioneering CERN experiments. ⁽⁹⁾ )

The three basic equations of the RLM are given in Figure 2. They represent Newton’s gravitational Law coupled with Einstein’s special relativity and with the de Broglie equation of quantum mechanics.

In addition to these three scientific giants, there are two more exceptional scientists/philosophers who are worth mentioning, i.e., Plato with his famous phrase “Everything consists of triangles” and his student Aristotle with his equally prophetic “The cyclic motion is the origin of everything”, both exactly confirmed today by the RLM geometry.

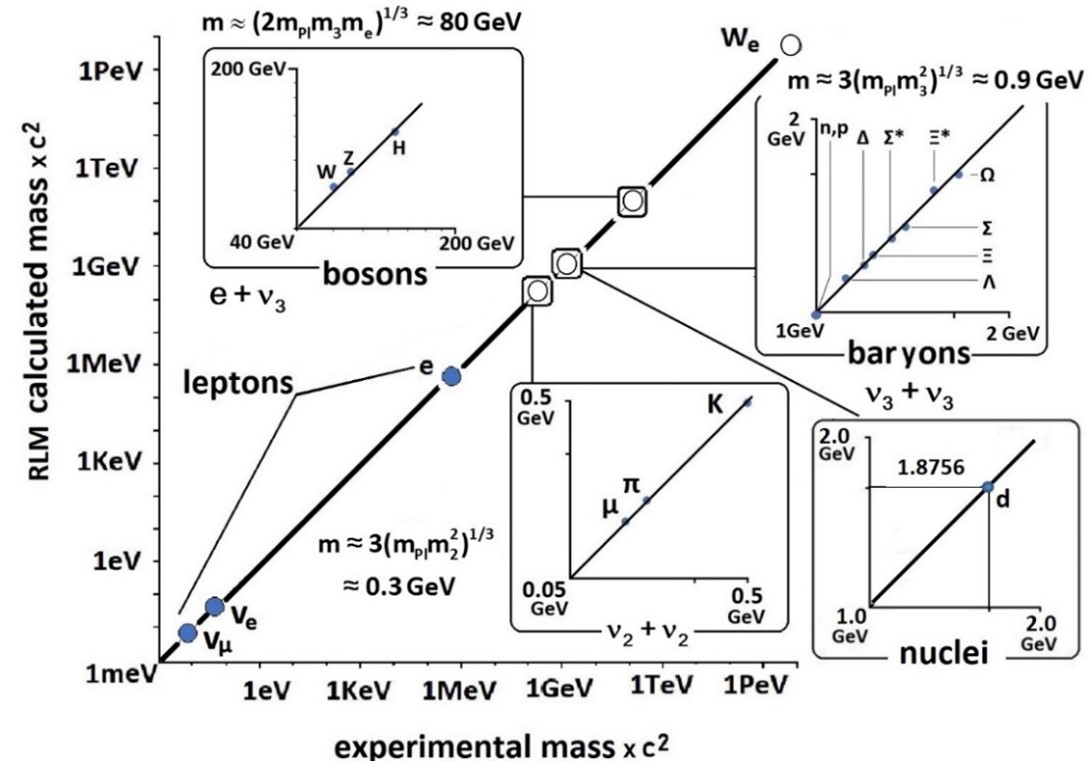
Figure 4 shows the geometries of the most common hadrons and bosons used to compute their masses via the RLM, while Figure 5 compares these computed masses with the experimental ones. ⁽⁶⁾ Agreement is typically better than 2%.

Gravitational catalysis

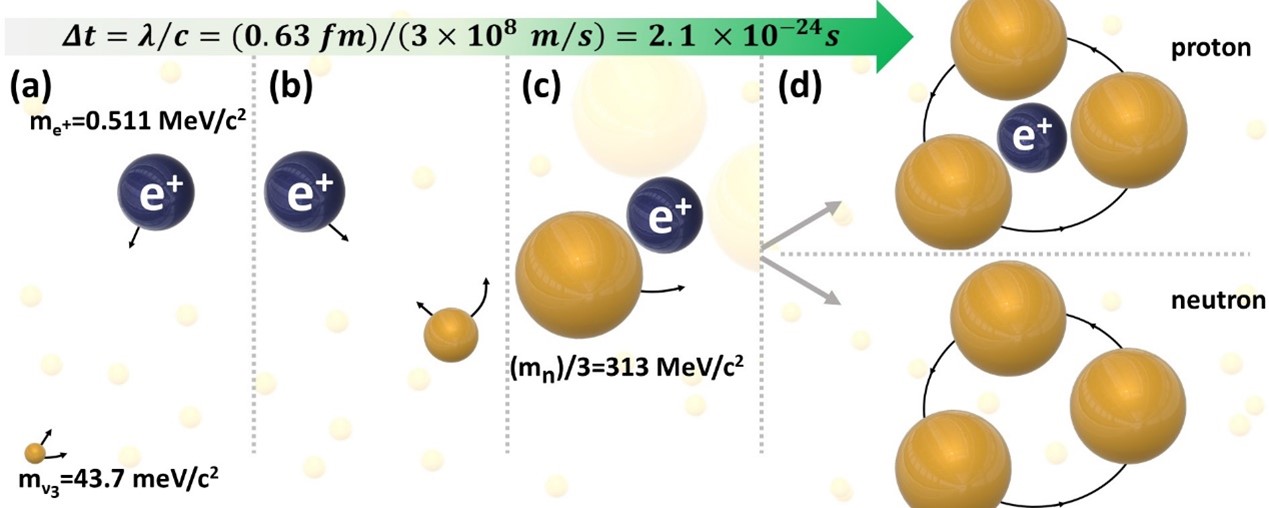
A basic question since the conception of the RLM is how the neutrinos reach or have reached the highly relativistic speeds which, via Einstein’s special relativity ⁽⁷⁾ bring their masses from rest masses (~10–2 eV/c2) (4,13) to the highly relativistic masses (~109 eV/c2) of those rotating in protons and neutrons. (12) The answer has been provided by some recent works ^(4,5,6), as shown in Figure 6. It results from an initial catalytic gravitational acceleration of neutrinos by positrons (and/or) electrons to highly relativistic velocities followed by the steady-state gravitational attraction by the other co-rotating neutrinos (Fig. 7). This two-step gravitational catalysis concept appears to be quite effective. ^(4,5,6)

References

1. Henso Abreu et al., First Direct Observation of Collider Neutrinos with FASER at the LHC, Physical Review Letters (2023).
2. R. Albanese et al., Observation of Collider Muon Neutrinos with the SND@LHC Experiment, Physical Review Letters (2023).
3. Takaaki Kajita, Nobel Lecture (2016): Discovery of Atmospheric Neutrino Oscillations. Rep. Prog. Phys. 69, 1607-1635 (2006).
4. C.G. Vayenas & S. Souentie, Gravity, special relativity and the strong force: A Bohr-Einstein-de-Broglie model for the formation of hadrons. Springer, New York (2012).
5. C.G. Vayenas, S. Souentie, A. Fokas. A Bohr-type model of a composite particle using gravity as the attractive force, Physica A, 405, 360-379 (2014).
6. C.G. Vayenas, D. Tsousis and D. Grigoriou, Computation of the masses, energies and internal pressures of hadrons, mesons and bosons via the Rotating Lepton Model, Physica A, 545 (2020) 123679.
7. A. Einstein (1905) Zür Elektrodynamik bewegter Körper. Ann. der Physik., Bd. XVII, S. 17:891-921; English translation On the Electrodynamics of Moving Bodies (http://fourmilab.ch/etexts/einstein/specrel/www/) by G.B. Jeffery and W. Perrett (1923).
8. A.P. French (1968) Special relativity. W.W. Norton and Co., New York.
9. R.L. Workman et al. (Particle Data Group) (2022) The review of particle physics. Prog. Theor. Exp. Phys 2022:083C01.
10. The Rotating Lepton Model: Electron and Positron Catalysis of Chemical and Nuclear synthesis, Open Access Government, October 2023. https://www.openaccess government.org/article/the-standard-model-sm-and-the- goal-of-force-unification-gravitational/150170/
11. A.S. Fokas, C.G. Vayenas. On the structure, mass and thermodynamics of the Zo bosons. Physica A, 464, 231-240 (2016).
12. D. Griffiths (2008) Introduction to Elementary Particles. 2nd ed. Wiley-VCH Verlag GmbH & Co. KgaA, Weinheim.
13. A.B. McDonald, Nobel Lecture: The Sudbury Neutrino Observatory: Observation of flavor change for solar neutrinos. Rev. Mod. Phys. 88, 030502(1-9) (2016).

Please Note: This is a Commercial Profile

This work is licensed under Creative Commons Attribution-NonCommercial-NoDerivatives 4.0 International.