The Nuclear Theory Group at the University of York, United Kingdom, develops novel theoretical methods for a precise description of ground and exited nuclear states, explained here by Jacek Dobaczewski, Chair in Theoretical Nuclear Physics
How do we understand the origins of matter in the universe? This overarching scientific question passes through the analysis of properties of atomic nuclei, which since the late 1930s have been tirelessly studied in increasingly sophisticated experiments. The database of precisely measured masses, radii, electric and magnetic moments, excitation energies, transition rates, decay lifetimes and many other observables are now enormous. The overall understanding of this data, developed in parallel with the experiment, is good.
However, is it good enough to declare victory? In fact, when it comes to details, systematics or extrapolations to uncharted territories, the precision of the quantitative description of nuclei is still far away from perfect.
Nuclear theory still has plenty of challenging questions to answer. In particular, only now can we begin to build approaches that have controlled uncertainties and can be systematically improved.
Why is this so difficult?
The fundamental theory of atomic nuclei is the Quantum Chromodynamics (QCD), which says that nuclei are built of two quarks, up quark and down quark, which interact by exchanging gluons. The QCD equations of motion are deceivingly simple to write down and exceedingly difficult to solve. We witness fantastic progress in this direction, mostly owing to the development of advanced algorithms and the employment of the biggest available computers. However, the prospects of deriving properties of heavy nuclei from such a fundamental approach are still beyond the horizon. The reasons for this are twofold. First, the lower the energy the more difficult QCD equations become to solve. Second, at low energies, those that characterise atomic nuclei, quarks and gluons cluster into composite particles: protons and neutrons. Therefore, although fundamentally the atomic nuclei are built of quarks and gluons, at low energies they look like they were built of protons and neutrons.
Nuclear Density Functional Theory (NDFT)
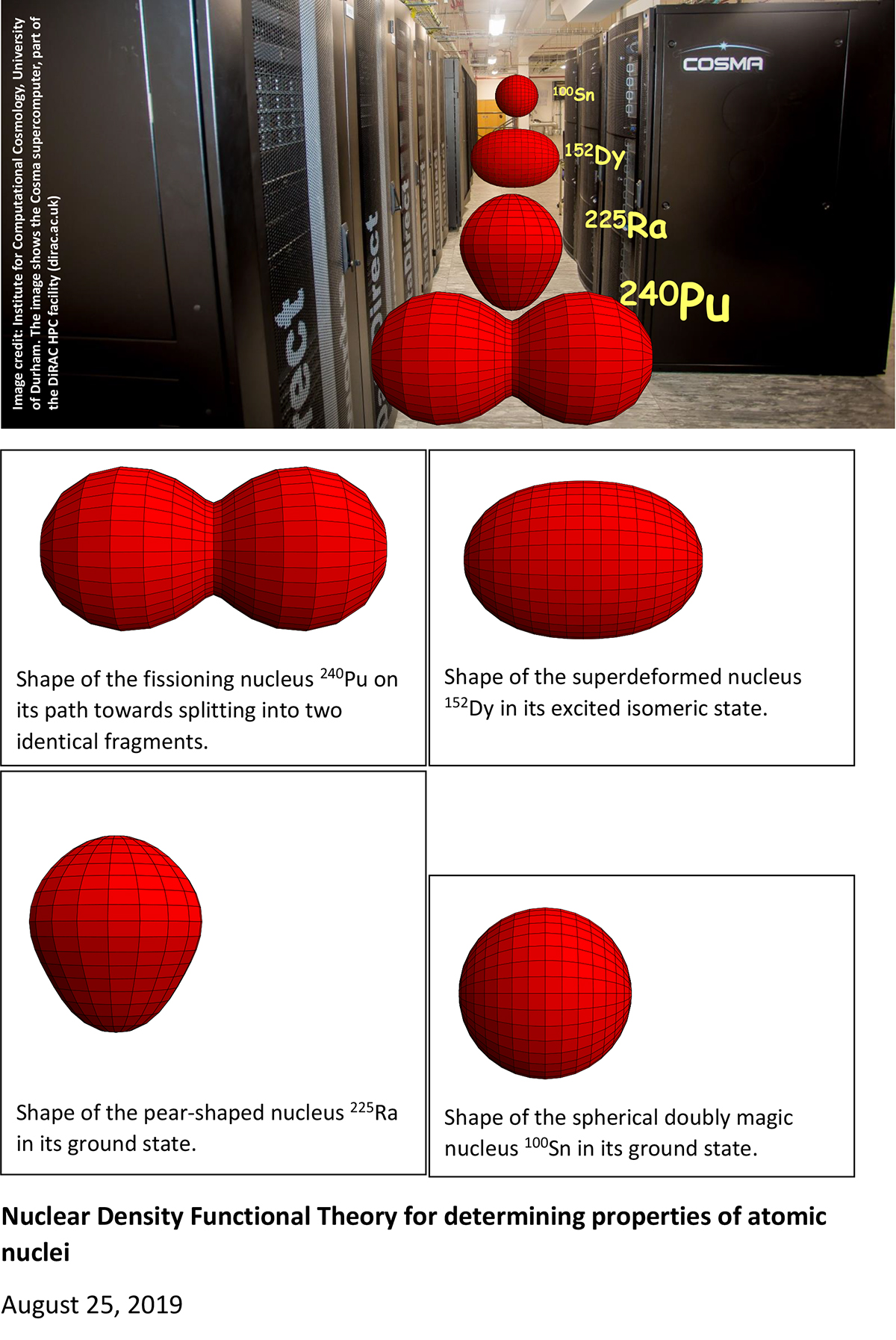
The NDFT makes one further step; namely, it assumes that at energies where we do not clearly distinguish individual protons and neutrons, we can limit the theoretical description to using their densities only. This simplification allows us to describe all nuclei very efficiently. Indeed, in its simplest possible version, the NDFT operates with two densities, the proton density and neutron density, instead of hundreds of individual protons and neutrons. In practice, realistic implementations of NDFT must take into account several other densities, for instance, their derivatives up to a certain order. Moreover, for many observables, we still must recall that individual protons and neutrons are visible. However, altogether we have at our disposal a consistent and manageable theory that properly describes complicated composite systems like atomic nuclei.
What do we really do?
Our long-term goal is to build novel sophisticated and precise NDFT that would be systematically improvable and would quantitatively describe low-energy nuclear properties with well-defined controlled uncertainties. To illustrate our present-day activity let me give you two specific examples. The first one pertains to applications of nuclear theory and to how it can contribute to societal and technological developments. It relates to the properties of one very peculiar isotope of thorium, 229Th, which has the first excited state at an unusually low energy level of 8.24 eV [1].
This energy is so low that the state can be manipulated with ultraviolet light. Typically, nuclear excitation energies are thousand or million times larger, so the excitation of a nucleus by ultraviolet light can be compared to steering a jumbo jet with a hairdryer. Using 229Th, it may be possible to build a laser-induced nuclear clock of unprecedented precision, which can revolutionise applications like an ultra-precise Global Positioning System (GPS). The NDFT will never be precise enough to describe such low excitation energy; nevertheless, it is perfectly applicable to determining electromagnetic transition probabilities, which will be essential for future laser applications.
The second example is our study of 225Ra [2]. This nucleus is the best candidate to measure hypothetical electric dipole moment (EDM) of the radium atom. Its unusual ground-state shape of a pear (see figure), favours detection of the parity-violating sector of strong interactions, which we were able to evaluate using our NEDF technology. If such non-zero EDM is ever measured, our calculations allows linking the experimental results to properties of parity-violating interactions and further, to a puzzling feature of our universe being built of the ordinary matter and not of the antimatter.
What does the future hold?
The nuclear physics studies that employ NDFT belong to the class of basic research that is curiosity-driven. It is the curiosity of what is around the corner that makes us tick. The history of science teaches us that basic research always leads to technological advances and improvements in human life. We do not know which part of it will, but it will undoubtedly. Teaching and training through basic research is the best way to form the minds of young people who then can do amazing things. The NEDF studies of atomic nuclei are an active area of basic research, giving us a possibility of extrapolating nuclear properties from stable to exotic systems and from terrestrial to stellar environments.
Finally, doing the NEDF research through one’s entire life is absolute bliss and fun.
Chair in Theoretical Nuclear Physics at the University of York, Professor Jacek Dobaczewski.
References
[1] Benedict Seiferle et al., Nature 573 , 243–246: Johannes Thielking et al., Nature 556, 321–325.
[2] J. Dobaczewski et al., Phys. Rev. Lett. 121 (2018) 232501.
*Please note: This is a commercial profile











